Tag: H-R diagram

Spectroscopic Parallax

For the nearest stars, the change in the position of the Earth in its orbit results in a tiny shift in the position of the nearby star relative to the distant background stars. This shift is called the trigonometric parallax. You can see the effect by holding your thumb up at arms length, closing your left eye, and lining up your thumb with something across the room. Now, alternate back and forth between having your right eye open and your left eye open and you’ll see the position of your thumb shift relative to an object further away. Move your thumb closer, and the shift is larger. That is the essence of trigonometric parallax.

Trigonometric Parallax

The distance to the star in parsecs (1 pc = 3.26 ly) is just

Now, a star’s distance, apparent brightness, and “true” (or intrinsic) brightness are related in the following way:

M = m + 5 (1 – log d)

where M = the absolute magnitude of the star

and m = the apparent magnitude of the star

and d = the distance to the star in parsecs

The absolute magnitude is the apparent magnitude the star would have if it were at a distance of 10 parsecs. Looking at it another way, the absolute magnitude is a proxy for the intrinsic brightness. The apparent magnitude is the star’s apparent brightness (as seen from Earth).

While the above equation is highly useful for general purpose calculations, to get the most accurate values astronomers must take into account atmospheric and interstellar extinction. And, anytime we deal with a star’s luminosity and its apparent brightness at some distance, d , we must specify the photometric system and optical filter that is being used. Or, less commonly (for practical reasons), we specify that the star’s luminosity and apparent brightness is to include all wavelengths of the electromagnetic spectrum, thus bolometric magnitudes are to be used.

Spectroscopic parallax is a bit of a misnomer, but here’s how it works for approximating the distance to main-sequence stars that are too far away to exhibit a measurable, reasonably certain, trigonometric parallax: measure the apparent magnitude of the star, and then using its spectrum to find its position on the H-R diagram, read off its absolute magnitude. Using your measured apparent magnitude and the star’s estimated absolute magnitude, you can solve for d the distance in the above equation.

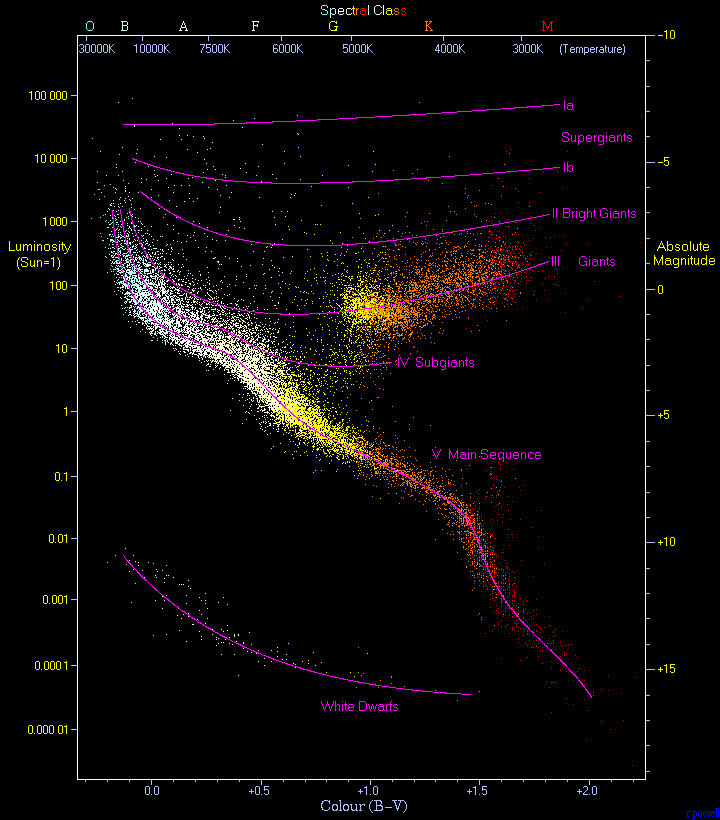
Hertzsprung–Russell (H-R) diagram

The star’s color (the x-axis on the H-R diagram) is easy to measure, but a deeper analysis of the spectral lines is needed to determine whether the star is a main-sequence, giant, or supergiant star (or something else).

Henry Norris Russell

Today, we celebrate the 140th anniversary of the birth of one of America’s greatest astrophysicists: Henry Norris Russell (1877-1957).  Called the “Dean of American Astronomers”, he is perhaps best remembered for his discovery of the relationship between the luminosity (absolute brightness) of a star and its color.  We call any plot of luminosity vs. color for a group of stars an H-R diagram, named after Russell and Danish astronomer Ejnar Hertzsprung (1873-1967) who independently discovered this relationship.

Russell noticed that cool (relative to other stars) red stars come in two varieties: those that are dim, and others that are very bright.  The only way a cool, red star could be so bright would be if the star were very, very large1.  In this way, Russell discovered that there are red giants and red dwarfs, but no medium-sized red stars.  Further studies by Russell and others led to the use of the H-R diagram as a tool in understanding the life cycles of stars.  Red giants, it turns out, are one of the final stages in the life of an ordinary star (like the Sun, for example).  Red dwarfs are low-mass stars that change very little throughout their lives.

After famously rejecting the revolutionary conclusion (in 1925) by Cecilia Payne-Gaposchkin (1900-1979) establishing that hydrogen is the primary constituent of the Sun and other stars, Henry Russell concluded four years later that Payne-Gaposchkin was correct, and acknowledged her significant contribution.  Moreover, he surmised that the main physical characteristics of stars are determined by just two basic parameters: mass and chemical composition.  This idea is known as the Vogt-Russell theorem, named after Russell and German astronomer Heinrich Vogt (1890-1968), who independently came up with the same idea.

An interesting sidenote.  Early in his stellar career, when he was just 24 years of age, Henry Russell wrote an interesting article published in the May 1902 issue of Popular Astronomy and dated March 24, 1902: “Shadows Cast by Starlight”.  It is a fascinating read—all the more special because it was written at a time (now over 115 years ago) when light pollution had not yet destroyed our nocturnal environment.

1Here we are comparing stars at comparable distances, such as in a star cluster.